Formel
für das
Skalarprodukt |
 Neue Formel für das Skalarprodukt
Um nun Praktisch mit dem Skalarprodukt zu rechnen,
wollen wir die Formel der Vorseite in einer etwas
anderen Form angeben: Neue Formel für das Skalarprodukt
Um nun Praktisch mit dem Skalarprodukt zu rechnen,
wollen wir die Formel der Vorseite in einer etwas
anderen Form angeben:
Das Skalarprodukt ist auch definiert
als:
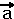 · ·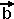 = | = |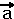 | · | | · |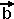 | · cos | · cos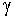
|
Wie kommt man auf diese Formel? Die alte Definition lautete:
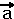 · ·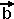 = | = |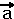 | · |Projektion von | · |Projektion von 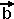 auf auf 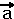 | |
Das zugehörige Bild sah so aus:
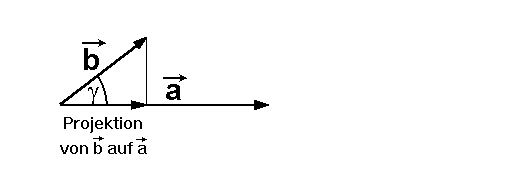 Den Betrag der Projektion von
Den Betrag der Projektion von 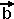 auf auf 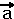 kann man einfacher kann man einfacher
ausdrücken, nämlich durch die Seite 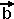 und den Winkel und den Winkel 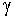 : :
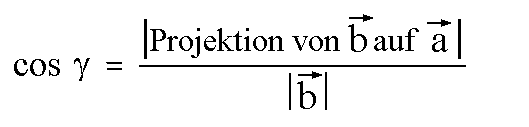 Diese Formel stellen wir um:
Diese Formel stellen wir um:
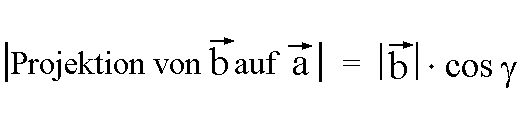 Die Formel setzen wir nun in die alte Definition des
Die Formel setzen wir nun in die alte Definition des
Skalarproduktes ein, und erhalten dadurch die neue Formel:
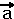 · ·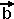 = | = |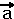 | · |Projektion von | · |Projektion von 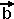 auf auf 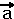 | = | | = |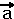 | · | | · |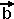 | · cos | · cos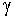
|